Single crystals of ternary semiconductor compound ZnGeP₂ with chalcopyrite structure are used as a high-performance medium for conversion of laser frequency radiation in the middle IR, what allows to solve a lot of problems of high-resolution spectroscopy. The crystals are also very promising for creation of sources of tunable THz radiation. Literature data about real ZnGeP₂ structure are practically absent.

In present contribution an analysis of structural defects in ZnGeP₂ crystals by X-Ray transmission topography for the first time has been carried out. ZnGeP₂ single crystals are grown by seeded Vertical Bridgman method from melt in Institute of Monitoring of Climatic and Ecological Systems SB RAS (Tomsk, Russia) and Single Crystal Growth Laboratory in School of Chemical Engineering, Harbin Institute of Technology (Harbin, PRC).

The state-of-the-art results in ZnGeP₂ growth with sufficiently perfect structure allow to register a presence of Borrmann effect and to apply X-Ray topography method based on this effect (XRBT method) [1]. The method has a high sensitivity to defects of crystal lattice and it has already shown its high effectiveness for a wide group of semiconducting materials such as Ge, Si, GaAs, SiC, and monocrystalline alloys of Bi-Sb [2]. The identification of defects by X-Ray transmission topography, based on Borrmann effect also shows that our ZnGeP₂ has a rather perfect structure, since only perfect crystals can be studied by this method. Additional methods in this work were method of topography in back reflection geometry and the method of photoelasticity (birefringence contrast method). Comparison of the information received by various methods of topography is spent.

It was studied the distribution of defects in the crystal volume, the factors affecting for distribution and density of dislocations was identified. Examples of topographs of cross-section and longitudinal slices of ZnGeP₂ ingots are shown on Fig. 1 and Fig. 2 respectively.

 Fig. 1. Example of topograph of cross-section cut of ZnGeP₂ ingot.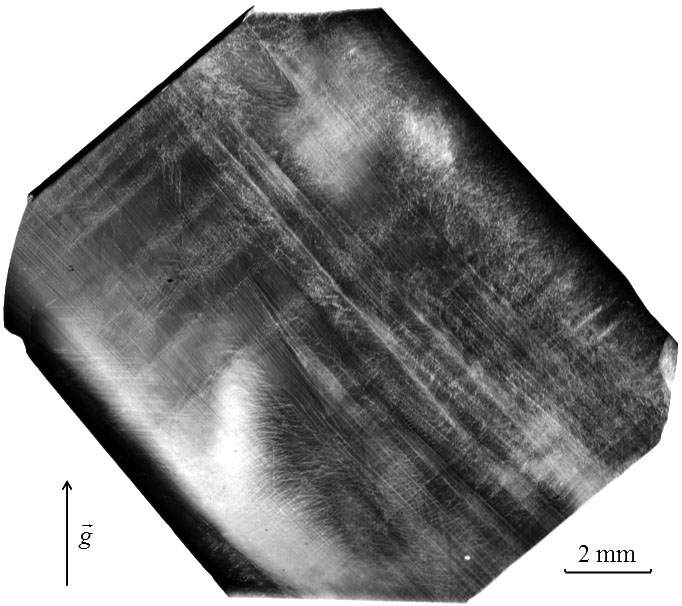

 Fig. 2. Example of topograph of longitudinal cut of ZnGeP₂ ingot.

By XRBT method images of all the main types of defects of crystal lattice – three-dimensional, or volume (large inclusions, dislocation networks, the elastic stress fields), two-dimensional, or planar (twins and stacking faults, Fig. 3), one-dimensional (dislocations of different slip systems), quasi-point defects (micro-inclusions of various types and small dislocation loops) were found and identified in ZnGeP2 crystals.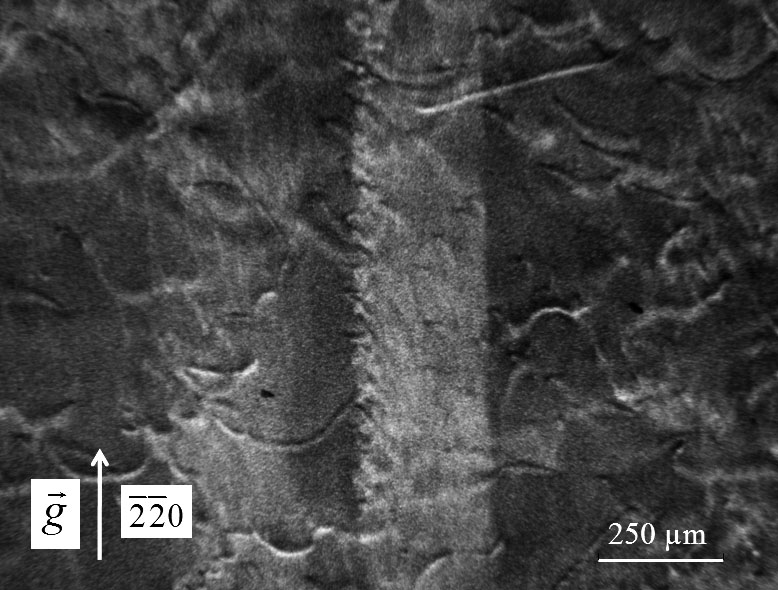

 Fig. 3. Magnified image of giant growth stacking fault in sample cut perpendicular to the growth axis of the ZnGeP₂ crystal.

In addition, the contrast from specific defects of this material – dislocations with strong impurity atmosphere and chains of inclusions, known as «solute trails» was observed also. Images of these defects were studied and described.

Under certain conditions, at the XRBT method defect is formed an image in form of contrast rosette with multiple petals. For example, the rosette is formed, if the axis of the dislocation lies in the reflection plane of the crystal along the direction of propagation of the energy of the X-ray wave field. Microdefect forms a rosette of contrast, if it located at a smaller distance from the output to X-rays surface of the sample than the "depth of vision" of the defect. Examples of images in the form of contrast rosettes from edge dislocations are shown in Fig. 4. Fig. 5 and Fig. 6 demonstrate examples of images of microdefects (small dislocation loops and microinclusions of second phase, respectively).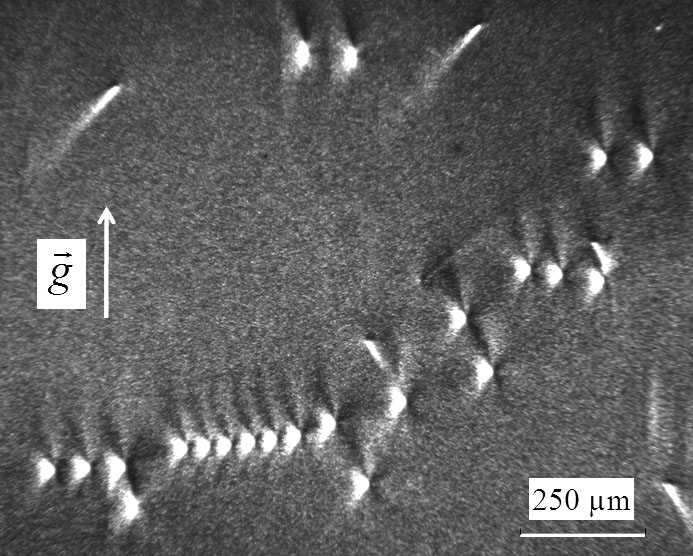

 Fig. 4. Rosettes of contrast from edge dislocations in ZnGeP₂.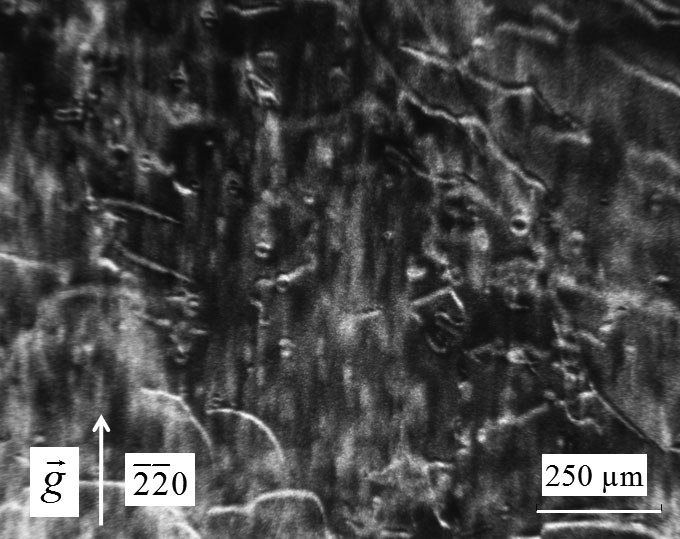

 Fig. 5. Images of small dislocation loops.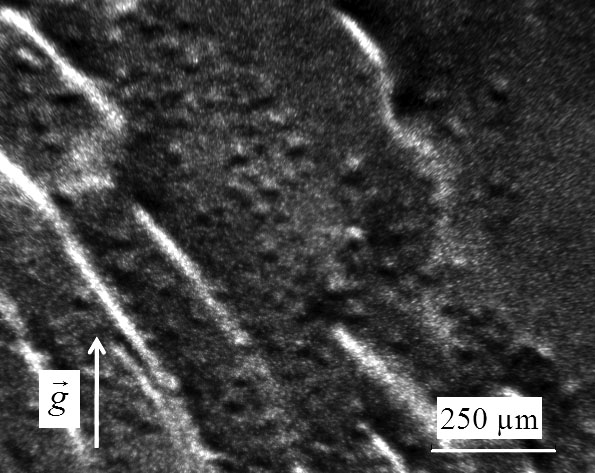

 Fig. 6. Topograph of a sample area with high density of "vacancy" type microdefects.

Identification of defects is carried out by comparing of their images on the experimental topographs with simulation ones or with already decoded images of defects. For the computer simulation a semi-phenomenological theory of contrast on the basis of Indenbom–Chamrov’s equations modified by L.N. Danil'chuk may be used. Recently the possibility of direct simulation of dislocation images in thick absorbing crystals by solving of Takagi equations was shown [3]. Images of "rosette" type for the same defect in different materials are similar, differing in size, proportions and the character of shape changes by varying one of the parameters (for example, at changing the depth of the microdefect location) [2]. XRBT method differs from other methods of X-ray topography due to higher informativeness and reliability. From the images in the form of contrast rosettes we can identify all main parameters of defects reliably and unequivocally: for dislocations these are direction of a dislocation axis, direction, sign and magnitude of the Burgers vector, and for microinclusions­ the type of lattice strain (microdefect of "vacancy" type or "interstitial" type), the power of the defect and the depth of its location in the crystal volume.

Obtained results show great possibilities of the "rosette" technique of topography in registration and study of structural defects in ZnGeP₂ crystals. A revelation of features of experimental topographs and their comparison with theoretically calculated will allow to create an atlas of experimental and calculated topographs of defects in ZnGeP₂, revealed by X-Ray transmission method based on Borrmann effect. This atlas in future should sufficiently simplify the study of real structure of ZnGeP₂ crystals.

This work was partially supported by RFBR grant № 12-02-00201.

1. A.O. Okunev, G.A. Verozubova, E.M. Trukhanov, I.V. Dzjuba, P.R.J. Galtier and S.A. Said Hassani // J. Appl. Cryst. (2009), Vol. 42, pp. 994–998.

2. L. Danilchuk, A. Okunev, V. Tkal, X-Ray Topography on Base of Borrmann Effect (LAP LAMBERT Academic Publishing, Saarbrücken, 2012), p. 348.

3. I.A. Smirnova, E.V. Suvorov, E.V. Shulakov // Fizika Tverdogo Tela (rus), 2007, Vol. 49, No. 6, pp. 1050–1056.

• Legal notice:
  

  Copyright (c) Pielaszek Research, all rights reserved.
  The above materials, including auxiliary resources, are subject to Publisher's copyright and the Author(s) intellectual rights. Without limiting Author(s) rights under respective Copyright Transfer Agreement, no part of the above documents may be reproduced without the express written permission of Pielaszek Research, the Publisher. Express permission from the Author(s) is required to use the above materials for academic purposes, such as lectures or scientific presentations.
  In every case, proper references including Author(s) name(s) and URL of this webpage: https://science24.com/paper/28764 must be provided.

1. Structural and optical properties of ZnGeP₂ single crystals
2. A High-power Generation in Mid-Infrared Region Based on ZnGeP₂ Optical Parametric Oscillation
3. Vertical Bridgman Growth of Large ZnGeP₂ Single Crystals for High Power Applications
4. Computer Diagnostics of Crystal Quality
5. The Analysis of Defects in ZnGeP₂ Single Crystals from Birefringence Images
6. Quantitative Criteria of Image Quality Evaluation, Theory and Experiment
7. Bulk Growth ZnGeP₂ crystals and their Study by X-Ray Topography