Introduction: ‘‘Storm glass’’ (also referred to as storm bottle, camphor glass) consists of a sealed glass tube containing five components of camphor, ethanol, water, ammonium chloride (NH4Cl), and potassium nitrate (KNO3) [1]. The pattern and quantity of the crystals change according to daily variation of weather condition and were empirically interpreted as a weather forecasting tool mainly in 19th century England.
Nakamoto et al. [2] examined the effect of several parameters of weather condition on the storm glass. The change in the appearance of crystals in the solution in the “sealed” glass tube was not caused by a change in atmospheric pressure, humidity, electric field, or magnetic field, but solely by a change in temperature. It was difficult to find a correlation between the variations in the crystallization and the changes in the weather useful in forecasting the weather.
In our previous study [3], the pattern formation of the crystals in the storm glass solution was investigated by focusing on the applied temperature. The main results are summarized as follows. (i) XRD showed that the crystals in the storm glass were camphor. (ii) Typical crystal patterns that appear in the storm glass were obtained using a directional growth technique by controlling the growth rates (cooling rate). (iii) The effects of temperature changes on the appearance of camphor crystals were observed. Two runs of temperature changes, i.e. cooling from 30 to 20 deg C and heating from 10 to 20 deg C showed the completely different appearance of the crystals even after keeping the final temperature of 20 deg C for 24 hours. The result suggested that the storm glass does not act as a thermometer, but rather an apparatus affected by the history of the temperature change. (iv) A cyclic temperature change between 30 and 20 deg C with the period of 20 hours was applied to the storm glass. Two runs for different initial conditions of 20 deg C with crystals and 30 deg C without crystals were compared. The height of the precipitated crystals repeatedly changed with the temperature change. The ranges of crystal height for two cases of initial conditions finally coincided after about five periods of the temperature cycle. The crystals in the storm glass were found to be affected by the history of temperature change over several days.
Kaempfe et al. [4] also showed that the ambient temperature had a major influence on the state of the weather glass (storm glass). A cyclic temperature change between two temperatures showed that the storm glass reversibly formed the same amount of crystals at a given temperature. It was concluded that the storm glass is chemically a complex system that is near the temperature-dependent solubility of camphor. A prediction of the weather was not possible.
Despite of the negative results of storm glass as a weather forecasting tool, we still have a strong interest in it. Generally, the crystals behavior under temperature variation has not been well characterized. Fundamental studies of growth mechanism and pattern formation mechanism of crystals have been carried out experimentally and theoretically under ideal condition, i.e. growth of a single crystal under uniform applied temperature. In this sense, there remain questions how we can predict appearance of aggregate of crystals and patterns of crystals under repeated temperature change. The study of the storm glass can be a model study of crystals (or a single crystal) behavior under complex growth condition.
Difficulties in understanding the crystal behavior in the storm glass lie in changing temperature accompanying growth and dissolution, multi-crystals interaction throughout the diffusion field of solute, and multi-component solution system. Our approach in the present study is simplification of the solution system. The objective is to clarify the effect of the components of the storm glass solution on the appearance of camphor crystals under cyclic temperature change. In addition, equilibrium temperatures of camphor crystal as a function of the camphor concentration in the solutions are obtained to estimate the amount of camphor crystals to discuss the appearance of the crystals.
Experimental: Three types of sample solutions are used; (a) Storm glass solution (five-component system: camphor 1.210g, ethanol 3.560 g, water 3.920 g, NH4Cl 0.300 g, KNO3 0.300 g), (b) three-component system (camphor 1.169 g, ethanol 3.727 g, water 4.100 g), and (c) two-component system (camphor 5.911 g, ethanol 3.266 g). The camphor concentration in the solution (weight ratio of camphor to the total weight of the solution) is (a) C = 13.0 wt%, (b) C = 13.0 wt%, (c) C = 64.4 wt%, respectively. The weights of camphor in the solutions are decided in order not to completely dissolve the camphor crystals at a highest temperature of the temperature cycle.
The experiment runs of cyclic temperature change were carried out as follows. The glass tubes (16.5 mm in diameter and 105 mm in height) with the different sample solutions were sealed and immersed in a programmable temperature controlled water tank. At an initial condition of 45 deg C, there is no crystal in all samples. The cyclic temperature change of a triangle wave function between TH = 28 deg C and TL = 21 deg C with the period of 10 hours is applied. The experimental run was continued for 48 cycles of temperature changes. The appearance of crystals (the height of aggregated crystals in the glass tube and crystal patterns) were recorded by a high resolution digital camera using an interval timer.
The equilibrium temperatures of camphor as a function of camphor concentration were obtained by microscope observation of the zero growth condition of a quite small single crystal in each solution in a thin glass cell. The temperature was controlled to an accuracy of 0.1 deg C using a thermo-electric module with a temperature controller.
Results and discussion: First of all, the images of camphor crystals in the glass tubes are shown. Fig. 1 shows typical images of crystals at TL = 21 deg C and at elapsed time t = 490 hrs. The numbers of components in the samples are (a) 5 components (storm glass), (b) 3 components (camphor-ethanol-water), and (c) 2 components (camphor-ethanol). Note that the height of the solution surface from the bottom of the glass tube is almost the same. The crystal heights in the samples (a) and (b) are twice as large as that in the sample (c). The crystals in the solutions of (a) and (b) look feather-like. Each crystal has dendrite pattern. The crystals in the solution of (c) look densely packed.
Fig. 2 shows the crystal height ratio to the solution surface vs the elapsed time. During the initial cooling, the crystal heights rapidly increased in all samples. The maximum crystal heights for 5 and 3 components are higher than that for 2 components. In case of 5 and 3 components, nucleated tiny crystals sank to the bottom of glass tube. Then the crystals with dendrite pattern grew upward. The crystal height ratios reached about 0.7 of solution surface. However, in case of 2 components, lots of tiny crystals repeatedly nucleated and sank to the bottom of the glass tube. The maximum height ratio resulted in 0.5 which is lower than the cases mentioned above.
In the process of the cyclic heating and cooling processes, the crystal height decreased and increased. Local maxima and minima of crystal heights for each sample gradually decreased repeating temperature cycles, and finally kept almost the constant values. It should be emphasized that not only the crystal height but also the amplitude of the height variation for 5 and 3 components are larger than those for 2 components.
The equilibrium temperatures , Teq, of camphor crystal as a function of camphor concentration were obtained (not shown). At the camphor concentrations of the three samples, the equilibrium temperatures are 31.1 deg C (5 components), 27.9 deg C (3 components), and 28.3 deg C (two components). Considering the temperature change applied, the weights, w, of the camphor crystals precipitated in the solutions were estimated simply assuming the equilibrium condition neglecting the concentration distribution in the solutions. For example, at TL = 21 deg C, the values of w are 0.30 g for 5 components, 0.20 g for 3 components, and 0.37 g for 2 components. The result showed that the values of w at TL in the solutions of 5 components and 3 components were smaller than that for 2 components, although the crystal heights as shown in Fig. 1(a) and 1(b) were much higher than that in Fig. 1(c).
In addition, at TH = 28 deg C the values of w are 0.11 g for 5 components, 0 g for 3 components, and 0.01 g for 2 components. The differences of crystal weight estimated, Δw, between TH and TL, is 0.19 g (5 components), and 0.20 g (3 components) which were much smaller than 0.36 g (2 components), although the amplitude of height variation in the solutions of 5 components and 3 components were much larger than that of 2 components. It should be noted that TH is close to the equilibrium temperatures, the local minima of the crystal height variation are much larger than 0. This might be the effect of increase in camphor concentration near the dissolving crystals in the solution.
Both results mentioned suggest that the porosities of the aggregate of crystals in the storm glass solution and the camphor-ethanol-water solution are quite high due to feather-like (dendrite) crystals. It is concluded that the large amplitude of height variation and dendrite pattern formation of crystals during the temperature change in the storm glass might be one of reasons that people in 19th century had an interest in the appearance of crystals in the storm glass in connection with the change in weather condition. The difference in crystal appearances between the storm glass solution and 3-component solution without NH4Cl and KNO3 was not clarified.
References:
[1] R. Fitzroy, The Weather Book, Longman, Green, Longman, Roberts, and Green, London, 1863, p. 439.
[2] Y. Nakamoto, M. Hiroi, Bull. Kobe Univ. Mercantile Mar. (Japanese) 38 (1990) 143.
[3] Y. Tanaka, K. Hagano, T. Kuno, K. Nagashima, J. Crystal Growth 310 (2008) 2668.
[4] P. Kaempfe, K. Molt, M. Epple, Chem. Unserer Zeit 46 (2012) 26. 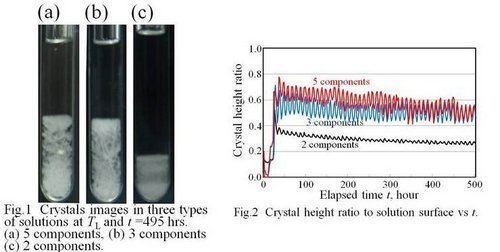 |


